The Triangular Sum Theorem states that the measure of the three interior angles of a triangle add up to 180 degrees. In geometry, that's basic knowledge!
The Triangular Sum Theorem
A visual project for chapter 4, section 1 of McDougal Littel's Geometry book. My Geometry final!
What is the Triangle Sum Theorem?
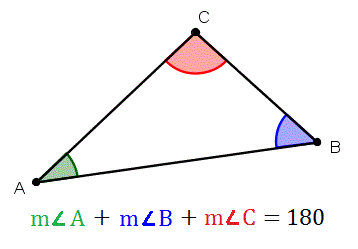
You may be familiar with the sum of quadrilaterals adding up to 360 degrees. For triangles, however, they only add up to half of that, as the Triangular Sum Theorem states.
How is the Triangular Sum Theorem Used in Real Life?
A good example of the Triangular Sum Theorem would be when constructing a house. The roofs of houses are often formed as a triangle. To get the measurements, you must know the degrees of all three interior angles.
Let's say that your two existing angles are 90 degrees and 45 degrees. To find the third angle, you may use the formula: a + b + c = 180. When you plug the values in and do the math, the third side of your triangle will be 45 degrees. Hooray!
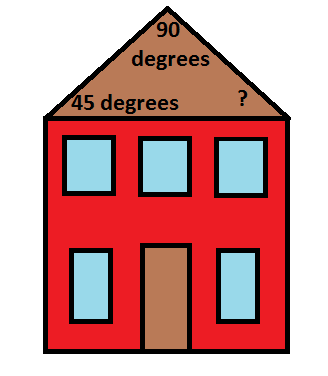
Careers that use the Triangular Sum Theorem are construction workers and roofers.
Why is the Triangular Sum Theorem Important?
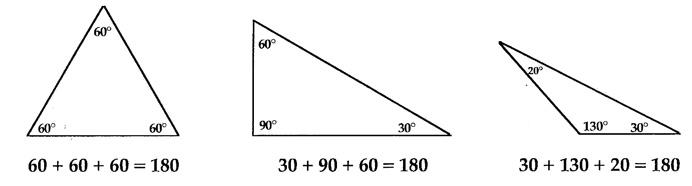
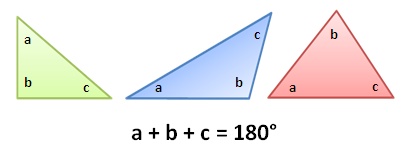
It is important that all of the interior angles of a triangle equal 180 degrees. In real life, a balanced triangle will have the interior angles equal enough to support the exterior. Despite sizes(right) and numbers(above), the Triangle Sum Theorem will apply to all of the interior angles of every triangle.
The Triangle Sum Theorem is also used in nearly every missing angle problem in geometry.
How Do You Prove the Triangle Sum Theorem?
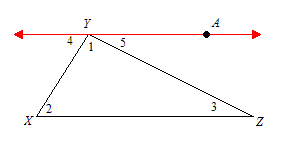
Since the theorem states that all three interior angles add up to 180 degrees, you will have to prove it. To the left, you are provided triangle XYZ.
To start, you need to construct an auxiliary line that is parallel to XZ through point Y. In the diagram, this point is labelled in red as AY. You will use the Angle Addition Postulate and the Linear Pair Postulate to add the measures of the angles.
To put it simply, substitute this equation: m<1 + m<5 + m<4 = 180. Easy, right? Now, you'll use the Alt. Interior Angles Theorem and the reasoning of Definition of Congruence to prove that the angles are congruent.
Finally, substitute. Use the simple equation: m<1 + m<3 + m<2 = 180. Now you have proof!
Here's a blank diagram to help you understand better.
Why Does this Theorem Only Apply to Triangles?
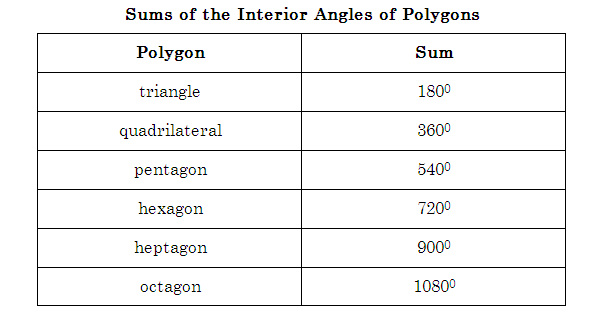
To see why the Triangle Sum Theorem only applies to triangles, compare it to other polygons using the chart on the left. The reason they add up to 180 is because of both the interior and exterior angles of the triangle. With polygons, they do not have 3 sides and will result in a larger sum of the interior angles than triangles.

